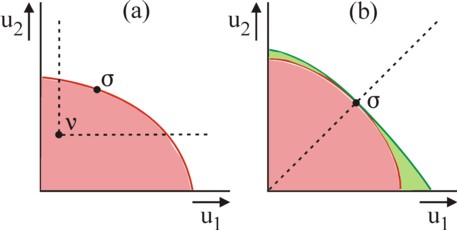
Figure 1: Sets of utility possibilities:
(a) status quo ν and solution σ;
(b) symmetrical set and expansion
The present column gives a detailed explanation of the axiomatic model of John Nash for the analysis of bargaining. Both the symmetric and the general variant are discussed. The model of Nash is associated with the model of Rubinstein for a negotiation with time pressure, with utilitarianism, and with the proportional method. It is shown, that from this Binmore derives a model of social morals. Finally the models are compared with experiences from practice.
The economic theory of the twentieth century mainly analyzes situations, where the distribution of wealth is given. In such a situation the social optimum can be realized by a paired exchange of goods between individuals, which is modelled graphically by the Edgeworth box. However, the Gazette has from the beginning also paid attention to the influence of group power to the social distribution. Incidentally, power itself is already relevant in the Edgeworth box, because it determines within the core of possible distributions which point on the contract curve is selected. Here and there in the Gazette references are made to marxism, which explains the distribution of incomes by the power, which originates from the property of capital goods. A distribution of income based on power is a bargaining process.
Naturally here a brief reference must be made to the scientific work of the marxist Sam de Wolff, the namegiver of the Gazette. He notably introduces an ingenious bargaining model, which wants to explain the distribution of the national income. According to De Wolff, the workers demand as their reward a fixed minimal utility1. Nowadays this minimum would be called the reservation utility of the workers. Next the enterprise can determine the working hours. He selects these in such a manner, that his profit is maximal. The model of De Wolff shows, that it is possible to mathematically model the bargain about the distribution. Here the negotiation is still one-sided, because in marxism the workers can merely enforce their own reproduction. After the Second Worldwar the mathematician John Nash has derived a morel general bargaining model, and this is the theme of the present column.
Various columns have applied the model of Nash, without explaining its theoretical foundations. A year ago the general Nash solution of a bilateral negotiation has been described, for the case of a network. Previously various columns have applied the model to the bargaining between trade unions and enterprises. Recently a model has been presented, where the state bargains with a firm about a subsidy. A more recent column relates the Nash solution to the social welfare function (SWF). So it is indeed time to study the Nash solution more closely. It has an interpretation, which deviates from the welfare functions. Namely, the SWF describes the preferences of a central planning agency with respect to the distribution. The Nash solution holds for the situation, where a neutral arbiter decides about the distribution problem. The conceptual difference is indeed marginal2.
Nash has developed his model for a bilateral transaction. However, it can be applied simply to larger groups. Suppose that the studied group consists of N individuals. The members of the group are together involved in a transaction or enterprise, which creates value. Let xk (k=1, ..., K) be the quantities of benefits k resulting from the activity. The group must compromise about the distribution of the benefits xk. Benefits are by definition useful. The preferences of the individual n are described by the utility function un(x1(n), ..., xK(n)), where xk(n) is the quantity, which is received by n. Each distribution leads to a utility vector u = (u1, ..., uN). The total of utility vectors u forms a set U of utility possibilities. In other words, the deliberations about the enterprise create the set U. The figure 1a shows such a set for the case N=2 (in pink)3.

The transaction only proceeds, when all N individuals agree. When no agreement is reached, then the existing situation remains intact. This utility point ν is called the status-quo. See the figure 1a. Loyal readers will recognize an analogy with the Edgeworth box, which has a similar starting point ξ, albeit of quantities of benefits xk. The set of utility possibilities obviously has a boundary. For, the quantities xk are also bounded. This frontier is represented by the utility possibility curve. It is shown in red in the figure 1a. Nash assumes, that the set of utility possibilities is convex4. Next he derives the solution σ of the distribution problem by proposing five properties, which could in reason hold for the wanted solution. This is called an axiomatic approach.
The five properties will each be discussed now. The first property is individual rationality. This is to say, the individual n will not accept solutions, which are worse than his status quo νn. In the figure 1a this property is represented by dashed lines for both individuals. The solution can not be below or to the left of the dashed line. An individual would indeed be foolish to cooperate in a transaction, which would only bring him disutility. Apparently νn is the reservation-value of n. For a singular transaction νn is the threat- or breakpoint. In an ongoing (continuously repeating) transaction, νn is the point of impasse or deadlock5.
The second property is the Pareto efficiency. The individual will only accept solutions, which are on the curve of utility possibilities. For, as long as this is not the case, he can improve his utility without hurting the utility of the other individuals. Note that the Pareto property requires, that all individuals dispose of complete information about the transaction. Namely, only then can they determine their maximally realizable utility. Suppose that the agreement is a (vector) contract σ. As an illustration the figure 1a shows this contract σ, located on the curve of utility possibilities. Note that the properties of individual reationality and Pareto efficiency are also revelant for the Edgeworth box. Here they define the so-called core6.
The third property is symmetry, also called anonimity. It holds for symmetrical sets U, as is shown in the pink area of the figure 1b. Such a set remains exactly identical, when it is mirrored in the 45o line coming from the origin (dashed in the figure 1b). The mirror-image is in fact a permutation of the individuals 1 and 2. Now the symmetry property states, that for a symmetrical set all σn are identical. In two dimensions σn is on the 45o line, with σ1=σ2. The interpretation is, that identical individuals have the same utility in the transaction. Nobody is discriminated.
The fourth property is the independency of irrelevant alternatives. Suppose that during the deliberations about the contract σ an event occurs, which is irrelevant for the contract. The status quo ν does not change. The event does result in an expansion of the set U, for instance with the light-green parts in the figure 1b. Now the property requires, that such an irrelevant expansion does not change the optimal contract σ. The requirement seems trivial7. The property can also be presented reversely. Suppose that originally the green curve of utility possibilies is valid. The optimal contract is σ. The possibilities in the green area are irrelevant for the contract σ. Now these possibilities are eliminated. Then in the new situation the contract will still be σ.
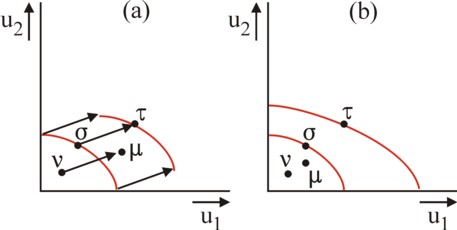
The fifth and final property is the independency of linear utility transformations. Such a transformation has the form u'n = αn×un + βn, where αn and βn are constants. So the transformation varies for each individual n. The explanation becomes more clear by considering the translation α and the relative scaling β separately. The figure 2a shows the translation. The whole utility field is simply shifted with respect to the frame of axes. The new status quo is μ. Since the utility to the left of ν1 and below ν2 is meaningless because of the individual rationality, the situation has not really changed. The new Nash solution is simply shifted in the same manner, and is τ. The independency of the translation is convenient, because ν can be shifted to the origin. This makes the problem more transparant.
Nash assumed an independency of the relative scaling β, because he wants to avoid interpersonal utility comparisons. For, the utility un is expressed in the utils (utility units) of n. When the contract σ would depend on βn, then the utility of the individuals n and m could be compared by means of the ratio βm/βn. Traditionally economists are reserved in this regard, because then actually a moral judgement would be made between the interests of the individuals n and m. Concretely, the independency for relative scaling β implies, that the scaling changes the contract σ in τ, with elements τn = βn×σn. See the figure 2b. This is to say, the old contract simply scales in the same manner as the utility field. Or more formally: let B be the scaling. Then the contract for B(U) with status quo B(ν) equals B(σ).
Now, by means of these five properties the Nash solution for a negotiation can be defined. It is a recipe for calculating the vector σ(U, ν), which represents the agreement between the N group members. In other words, σ is determined by the set U of utility possibilities, the status quo ν, and the recipe. This recipe is:
(1) maximize for the vectors u in U: Πn=1N (un − νn)
In the formula 1, Π is the mathematical symbol for multiplication of the N terms. Nash discovered, that this solution satisfies the five mentioned properties. Moreover it can be proved, that the recipe of the formula 1 is the only (unique) solution8.
The following notes concerning the formula 1 are worth mentioning. Actually the five properties have more resemblance to an arbitrage (judgement about a conflict) than to a negotiation. This has already been remarked in the introduction. It is supposed, that the N individuals cooperate. In particular it is ignored, that they could mutually form coalitions in order to obtain a better result for themselves than the Nash solution. Incidentally, this was not relevant for Nash, because he studied the case N=2. Furthermore, the Nash solution is not fair in the sense, that the status quo ν is accepted as fixed. And the status quo could be extremely unfair. The injustice in the starting position is also found in the similar model of the Edgeworth box. In particular, the model of Nash is not a recipe for maximizing the social welfare9.
The reader must be aware, that the independency of linear utility transformations has radical consequences. For instance, it allows to make the status quo ν invisible by means of a translation to ν'= 0. The Nash method indeed considers merely the differences un − νn. Besides, the maximally realizable un for all individuals n can be made identical by means of the relative scaling, for instance to unmax = 1. Then the difference between the individuals n is determined merely by the form of the boundary of the set U. It is intuitively logical, that a (qua utility) wealthy individual n with a strong status quo ν
In practical cases it is often necessary to give up the third property, the symmetry or anonimity. Then a parameter γn≥0 is added to the model, which characterizes the individual n. This modelling is called a general Nash negotiation. The general recipe to find the Nash solution is:11
(2) maximize for the vectors u in U: Πn=1N (un − νn)γ(n)
Loyal readers will observe a resemblance between the formula 2 and the individual utility function in the rational choice theory, which has the form u = Πm=1M cmγ(m). In the utility function, γm represents the weight, which the individual attaches to his need to own a quantity cm of the good m. In the same way γn in the formula 2 expresses the weight, which the arbiter or referee attaches to the interests and needs of the individual n, but now in comparison with the other individuals j≠n of the group.
As an illustration of the general solution a special case can be considered, namely a transaction with N=2, where the set U of utility possibilities is given by u1 + u2 ≤ π 12. This is to say, thanks to the transaction a quantity utility π can be distributed. It can be shown, that in this case the most reasonable contract along the lines of Nash is given by13
(3) σn = νn + (π − ν1 − ν2) × γn / (γ1 + γ2)
Here one has n=1 or 2. Since the solution does not depend on translations, one may assume ν=0. Then the individual n apparently obtains a fraction γn / (γ1 + γ2) of the available utility π. For this reason γn is sometimes interpreted as the bargaining power of n. This would then be the power, which the arbiter gives to the individual n. In the case of equal power the distribution is fair: σn=π/N.
Worth mentioning is the model of Rubinstein, which derives the general Nash solution in a totally different way14. This model is part of game theory, and is called the game of alternate offers. The transaction concerns a group of two members (N=2), and produces a total utility of π 15. The two individuals make alternate offers for the distribution of π. Note that they are both egoistically, and therefore do not aim at a just solution, like the arbiter. Therefore this is called a transaction without cooperation (although the individuals must naturally complete the enterprise together). Each time after an individual has made an offer, the other withdraws for a period Δt in order to consider the offer. The negotiation has a certain time pressure, because the individuals prefer to benefit from their utility as soon as possible. Therefore the utility is devalued with time, with a discount factor δn per period Δt.
Suppose that it is the turn of individual n to make an offer. Each rejected offer reduces the value of the transaction, because hereafter a time Δt will pass. Therefore n (=1 or 2) wants to make an offer, which is acceptable for the other individual m≠n. The individual n calculates, that it is optimal for m to offer a fraction xn of the total value to n. Then m himself obtains a fraction xm = 1 − xn. However, since m will make his offer later, the value of the transaction has again been reduced by δm with respect to the present. The individual n can make a convenient use of this devaluation. Namely, he offers to m a fraction δm×xm. This offer is precisely worth as much as the offer, which m will do a period Δt later. This is to say, m is indifferent with respect of these two offers. Therefore m will agree with the present offer. And n obtains a fraction xn = 1 − δm×xm.
Suppose that n by presumption or laxness makes an offer, which is too low. Then it is m's turn to make his best offer. The presented argument is also applicable to m. This is to say, his best offer is δn×xn. A rational n will agree, so that m gets a fraction xm = 1 − δn×xn. Substitute this formula in the found expression for xn, then the result is16
(4) xn = (1 − δm) / (1 − δn×δm)
Here one has of course δn×δm=δ1×δ2. The formula 4 is based on the assumption, that n can make the first offer. This gives him an advantage. Assume for the sake of convenience, that δ1=δ2=δ holds. Then the formula 4 becomes identical to xn = 1/(1+δ). Therefore m gets a fraction xm = 1 − xn = δ/(1+δ), so a factor δ less than n.
The discount factor δn and the discount rate dn are related by means of δn = 1/(1+ dn). Let rn be the discount rate, which represents the continuous devaluation, namely the devaluation for extremely small time intervals. Now it can be proved, that one has δn = exp(-rn × Δt) 17. Substitute this relation in the formula 4. It can be shown with the obtained formula, that in the limit Δt→0 one has xn = rm / (r1 + r2) 18. Here one obviously has n=1 or 2, n≠m, just like before. So this distribution xn occurs for offers with very small periods in-between. To be more precise, it can no longer be assumed, which individual makes the first offer. The distribution is always the same, irrespective of who begins19. For the comparison of the solution of Rubinstein with the Nash solution the following form is more convenient:
(5) xn = (1/rn) / ((1/r1) + (1/r2))
A comparison with the formula 3 shows, that the distribution xn from game theory is identical to the solution σn of the general Nash negotiation, under the condition that 1/rn = γn holds! According as the individual n has a smaller continuous discount rate rn, he gains power in the general Nash negotiation. This has some logic, because an individual with a small rn is slow in devaluing the value π of the transaction. Such an individual is patient. Apparently patience leads to power20. Furthermore, note that a long-lasting deadlock would have the consequence, that the transaction loses all of its value, due to the discount factors. Here the deadlock can be interpreted as the status quo. The breaking point consists of the external alternatives of the individuals. Here they are irrelevant, because the model of Rubinstein offers a certain solution21.
In the paragraph about the Nash solution the approach has been called axiomatic. The axioms lead to the Nash function fN(U, ν) in the formula 1 22. Although the five axioms of Nash are logical, one could also invent other axioms. Alternative axioms lead to another function f(U, ν). Certainly when fN is interpreted as the utility function of the arbiter, it is logical to search for alternative functions, which are also social welfare functions23. The utilitarianism and the principle of proportionality lead to well-known welfare functions, namely
(6a) fU(U, ν) = Σn=1N αn × (un − νn)
(6b) fP(U, ν) = minimum of αn × (un − νn) for n=1, ..., N
In the formula 6a the term -Σn=1N αn × νn is commonly ignored, because it is a constant. Welfare is always relative. But it turns out that in the present argument the notation of the formula 6a is convenient. It is immediately clear, that neither the utilitarian nor the proportional approach satisfies the independency of linear utility transformations. They also do not satisfy the property of symmetry (anonimity). On the contrary, the weighing factors αn discriminate between individuals, and in the formula 6b νn also discriminates. Therefore neither fU nor fP are sound functions for modelling the bargaining. Nevertheless they are important for the present column, because they have a special relation with the model of Nash.
Namely, the maximization of fU(U, ν) and fP(U, ν) commonly leads to two different optima on the curve of utility possibilities U. However, consider the special case, that both functions yield the same optimum σ for the welfare. Equate the value of the function fU(σ, ν) in the point σ to Wσ. By definition in the proportional optimum one has, that all αn × (σn − νn) have identical values. Thus one finds, that the common point σ must satisfy
(7) σn = νn + Wσ / (N × αn)
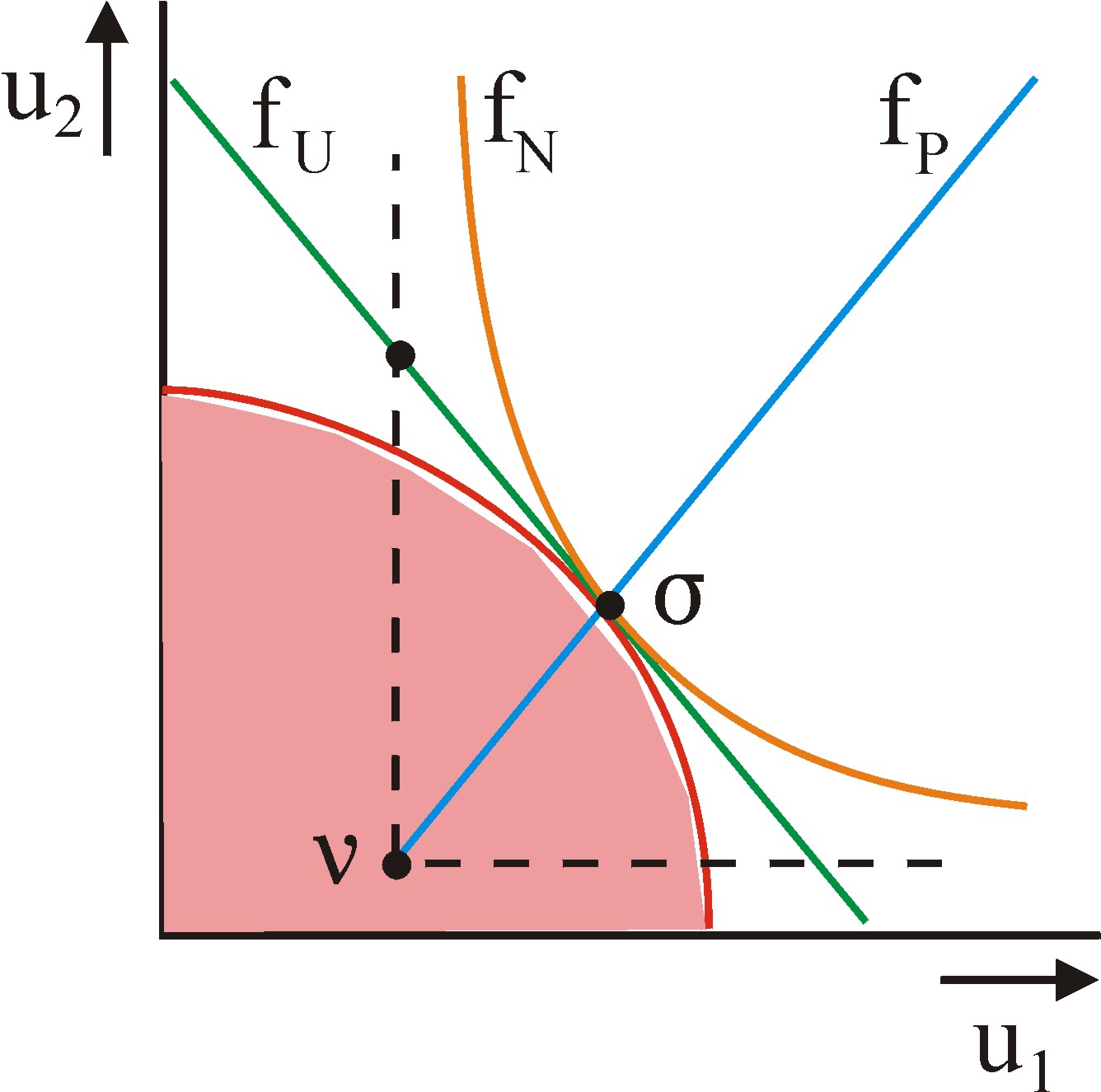
As an illustration the figure 3 shows the coincidence of the two optima for the case N=2. It strongly resembles the figure in another recent column, about welfare economics. The green line represents the function fU, and the blue line shows the relation between u1 and u2 according to fP. The slopes of the lines have the same absolute value, namely α1/α2, but it is negative for fU. The intersection of the two lines naturally is the point σ, located on the boundary of U. Note that the line fU through σ also contains the point u = [ν1, ν2 + Wσ/α2]. So in this point u2 − ν2 is exactly two times as large as σ2 − ν2, this is to say, u2 − σ2 = σ2 − ν2 24.
The case of a shared welfare optimum σ in utilitarianism and the proportional method has the typical property, that σ is also the solution of symmetric Nash bargaining (so with γn=1 in the formula 2)! This can be shown in the following manner25. First note that the Nash function fN(U, ν) in the formula 1 can be rewritten as
(8) gN(U, ν) = Σn=1N ln(un − νn)
For, gN = ln(fN) is maximal for the same u as fN itself. Furthermore note, that the formula 7 can be rewritten as αn = (N / Wσ) / (un − νn), calculated in the point u=σ. This is to say, αn = (N / Wσ) × ∂(ln(un − νn))/∂un, in u=σ. According to the formula 8, this is identical to αn = (N / Wσ) × ∂gN/∂un, in u=σ. Now the vector α is on the one hand the normal of the hyperplane, defined by fU=Wσ 26. On the other hand, the vectorial gradient ∂gN/∂un is the normal of the (N-1)-dimensional surface (set, if desired) gN(u, ν) = V, where V is a constant27. Therefore the surface gN(u, ν) = Vσ, which contains the point σ, must touch the hyperplane fU=Wσ, where σ is the only shared point. But then gN(u, ν) = Vσ touches the set U in σ. Apparently σ is indeed the point of U, which makes gN and fN maximal. Because of the formula 1, then σ is the Nash solution of the transaction. The orange curve in the figure 3 shows fN(u, ν) = exp(Vσ) for N=2.
The identification of the Nash solution with the utilitarian and proportional solutions with identical weighing factors α has a peculiar consequence, which is not stated clearly in the consulted sources. Namely, the Nash solution assumes the property of symmetry (anonimity). However, the utilitarian and proportional solutions empathically do not satisfy this property. Apparently the restriction of a proportional distribution removes the interpersonal comparison from the utilitarian welfare, and conversely. Nevertheless, this shows that the Nash solution can indeed be interpreted as a result, which is obtained by means of moral reflections, based on interpersonal comparisons28.
An important incentive for analyzing the transaction model of Nash is to gain more insight in the model of society of Ken Binmore, which is completely based on the Nash model. Half a year ago it has been explained in a column, that Binmore attributes the development of morals to the constitutional deliberations in the sense of the philosopher J. Rawls. The social contract is determined in deliberations of all citizens, which are done behind a veil of ignorance. Binmore states, that the veil filters the social position out of the memory of each citizen. However, the transparency of the veil is sufficient for observing the status quo ν of society. The deliberations can be modelled with the model of Nash. The citizens defend their own interest, but they are also capable of empathy. They can imagine themselves in the situation of others. This is essential for living together.
In a column of a month ago it is explained, that Binmore in his model of society makes a convenient use of the coincidende of the solutions of Nash, utilitarianism, and the proportional method (which is a variant of the maximin principle). Ordinary negotiations and the constitutional deliberations both follow the recipe of Nash. During the deliberations there is anonimity (symmetry), so that a moral judgement of interests is in danger of being omitted. However, this deficit is corrected by relating the Nash solution σ to utilitarianism and the proportional method. For, the weighing factors αn are a moral judgement about the manner of distributing the yield among the individuals. Thanks to empathy and the veil the deliberation ends in an agreement about the optimal values of αn. Binmore calls the αn the empathic preferences with regard to the various social positions n.
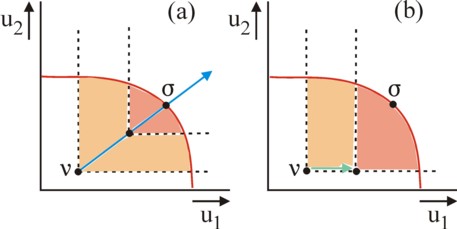
Since the deliberation about the social contract is a rare event, in the short run the αn values are fixed29. Apparently then the values of αn are in equilibrium. They can be adapted in the medium term, during the various constitutional deliberations. In the way the social changes can be taken into account. Then the interpersonal utility comparison αn/αm with n≠m is adapted to the new status quo. The various utility units (utils) get a different value. So the equilibrated morals evolve in a path-dependent manner. During the deliberations the morals are based on the utilitarian welfare function (formula 6a)30. For, there is a desire for a high and increasing welfare. The utilitarian solution is an expression of collective empathy. The deliberations must finally lead to consensus about a future society σ, and a plan (agreement) to realize it.
This social contract is only useful, when the growth path from ν to σ is feasible. The completion of the growth requires a certain time. This is daily life in the short term, where the individuals defend their own interest. Therefore they demand the application of the proportional solution. But then the growth to σ must follow the line through ν and σ, with in each point the same αn × (un − νn) for all N. See the figure 4a. In other words, the economic growth will by definition shift the status quo ν. When this shift does not follow the mentioned line, then the citizens will demand another Nash solution σ. They would like to renegotiate the contract. See the figure 4b31. Therefore the proportionality is essential. Thus the feasibility imposes a restriction upon the utilitarian maximization. This guarantees that the social contract becomes self-enforcing or self-policing (internalized).
Thus it seems that the scientific merit of Binmore is mainly, that he gives a social meaning to the existing scientific models. The ideological starting point is the image of man as an empathic egoist. In the status quo the individuals together conclude a social contract (constitution), which mirrors the collective morals. The individual empathic preferences are sufficiently similar for realizing a collective compromise. The result is the Nash solution, a compromise between welfare and justice, here in the sense of reciprocity. Binmore places the interpersonal utility comparison at the centre, as the basis for subjective ethics32. Then the proportional approach obtains the meaning of the individual self-commitment to the contract. In this manner Binmore presents an interesting abstract frame of thinking. However, your columnist does not know any practical applications of the model of Binmore33.
It is obviously important to know whether the model of Nash agrees with the practical experiences in the real world. The behavioural economics has studied negotiations in laboratory experiments. In such experiments it turns out, that the individuals interpret the available information in a biased manner (self-serving bias)34. This is evidently not rational. In such situations an arbiter can correct the views of the concerned actors. The model of Rubinstein has also been simulated in experiments. It turns out that in repeated games some individuals indeed learn to apply the formula 4. However, the learning process can be slow35. Apparently, at least in such experiments the assumption of rationally acting individuals is doubtful.
The symmetrical model of Nash ignores the influence of individual power. But often power will precisely be a decisive factor for the distribution. In the rational choice theory power is equated to the total value of the resources, which are available to an individual. In a previous column about this theory it has been stated, that wealthy individual can reinforce their position during a transaction by "buying" support. They have a lot to offer to the individuals in their network. Also beyond the rational choice theory the economic theories give a prominent place to power, such as the models of rent seeking. The role of power in the model of Binmore is difficult to interpret. One wonders, why a powerful individual would want to forget his position during the deliberations behind the veil36. On the other hand, power is perhaps indirectly included in the model of Nash, by means of the form of the boundary of the set U.
Furthermore, there is an enormous amount of manuals, which give advise about the strategy in negotiations, A special mention deserves the book Excellent onderhandelen (in short EO), which originates from the Harvard Negotiation Project, and therefore has a scientific foundation37. The authors emphasize the importance of the best alternative to a negotiated agreement (in short BATNA). Since the BATNA is simply the status quo νn, thus the model of Nash is confirmed. For instance, νn can be the utility value, which the individual n would obtain, when an external arbiter or court would decide about the distribution of the surplus. In EO it is emphasized, that the participants in the negotiations about the transaction must try to improve their status quo (breaking point, security level, reservation utility), so that νn is actually dynamic.
Another advise of EO is to study the mutual interests, because this can probably increase the available yield of the transaction. Then the set U expands. This is also called the win-win strategy. Showing empathy is a desirable property. In this regard, EO joins the view of Binmore. It also recommends to base the negotiations on objective criteria (costs, norms, conventions, scientific ideas, and the like). This increases the chance of a rational result. And the chosen solution must not be affected by irrelevant factors. In particular, EO points out, that the good relation between the individuals must be separated from the agreement. Thus various properties of the Nash solution are addressed in EO.

But there are also differences between EO and the model of Nash. Notably, EO absolutely does not assume symmetry or anonimity for the various participants in the negotiation about the transaction. A skilled negotiator gets better results than emotional or rigid individuals. The individual power, such as having an attractive BATNA, partly determines the distribution of the surplus. This criticism on the model of Nash obviously also holds for the model of Rubinstein. On the other hand, it must be acknowledged that an asymmetric situation will probably be rare in institutional bargaining, such as between pressure groups.
Among the Dutch publications, the book Onderhandelen (in short OH) has gained some prestige38. It argues, that bargaining requires a behaviour, which mixes cooperation and competition39. The arbiter in the model of Nash, who carefully weighs all interests, precisely suggests, that cooperation is desirable. In OH the bargaining is presented as a pre-eminently dynamic process, where each individual exerts influence on the balance of power, the prodedures, the atmosphere, and the personal rank-and-file. There clearly is not a situation of individual anonimity. According to OH, it is true that the negotiator must be prepared to compromise, but yet he must defend his own interests (un). This requires a behaviour, varying between exploring and remaining unrelenting. The exchange of information is rarely complete, because it is used in a tactical manner. Therefore the set U of alternatives is never known completely.
The conclusion of the model of Rubinstein is generally supported by the experts. For, the importance of patience is mentioned in all manuals about bargaining40. This literature confirms, that the available information is never complete41. The behaviour during the negotiations can be irrational42. Sometimes the win-win strategy is propagated43. Although the importance of the BATNA (ν) is acknowledged, yet the general view is, that it must always be attempted to break the deadlock44. Deadlocks are simply a natural part of the negotiation.
It is curious that little attention is paid to the true execution of the agreement45. For, Binmore rightly points out, that a contract is fragile, as long as it insufficiently meets the interests of the concerned individuals. A good agreement must encourage the self-commitment. The concerned group as a whole must be dedicated to its observance. And finally, especially the popular literature hardly distinguishes between political-institutional and daily bargaining46. So it is logical that it differs somewhat from the models in this column, which probably mainly aim at institutional processes.