Models of power

First insertion on Heterodox Gazette Sam de Wolff: 15 july 2017

Group power plays an important role in economic systems. The present column presents three models of the abuse of power. First, the power distribution in networks is analyzed. It turns out that in bilateral exchange processes the power is determined by the position in the network. Next, the theory of rent seeking behaviour by interest groups is addressed. The goal is to redistribute the incomes. Finally, the theory of the tournament as a source of rewards is studied. The tournament can increase the motivation of risk-neutral workers, such as management.

Since its foundation, the Gazette has given a central place to power factors within the economy. Your columnist has always doubted, that human actions would be coordinated mainly by the invisible forces of the markets, such as is stated by the neoclassical paradigm in its traditional form. Social power is always exerted by and within groups. At the end of the nineteenth century, the marxist theory of power has made a lasting impression. However, it was always controversial, and nowadays is generally rejected¹. Since the seventies of the last century the analysis of group power has become more popular, also among economists. The new findings are often presented under the name new institutional economics or new political economics.

The group uses her power in order to further het own material and immaterial interests. Power is used for exerting influence on others, in such a manner that they adapt their behaviour in the desired direction. For instance, power can be mobilized for spreading a certain religion among the people. The group mentally benefits from this, because each conversion is an affirmation of the personal identity. However, economic theories of power mainly place the material interests in the centre. Then the power aims to increase the welfare of the personal group. The present column describes several of the most popular theories of power.

Power in networks

The distribution of power naturally depends on the social relations between the various actors. The Dutch economist P. Frijters has proposed a taxonomy, which distinguishes between (loose) networks, reciprocal circles and hierarchies. This taxonomy is related to the categories, which have been proposed by institutionalists such as O. Williamson. At the micro level the bilateral or dyadic transactions between actors j and k in a network can be studied. An important approach is the so-called general Nask solution of the negotiation between j and k. The starting point is that thanks to the transaction the pair j and k can mutually share an income π². Define x_jk as the resulting income of j in this transaction with k. This is the reverse for x_kj. The rational solution of the exchange is given by

(1)     max_{x(jk), x(kj)}    x_jk^bj × x_kj^bk
         under the condition x_jk + x_kj = π

In the system 1 b_j is the total bargaining power of j, and b_k is the same for k. The solution of this problem is x_jk = π × b_j / (b_j+b_k), and evidently x_kj = π − x_jk. The value of b_j depends on the type of the transaction. It is logical that b_j will increase with the number of possible contacts n_j of j with other actors, where also the total number of actors in the network is relevant (j, k ≤ n). Furthermore, the number of mutual connections or ties m in the network influences b_j, because not all pairs of actors necessarily have a contact. In practice it turns out that it is convenient to combine n and m in the parameter w = (n+m) / (1 + n+m). The parameter w expresses, that in small networks j does not have much choice. And finally, the number a_j of transactions of j is important, because during a time interval (period) j can conclude various transactions with his n_j contacts³. Thus one has b_j = b_j(n_j, w, a_j).

In order to make b_j more concrete, the position of each of the n_j contacts in the network must be determined. This fills in the structure of the network. Suppose that the variable δ_jk indicates whether k has a contact with k, and in such a manner that δ_jk=1 represents a contact. Pairs without contacts, as well as δ_jj, are presented by the value 0 in the matrix Δ. This Δ can be used to derive the convenient matrix R, with elements ρ_jk = δ_jk / Σ_i=1ⁿ δ_ik. Apparently, ρ_jk measures the importance of this contact for k in relation to all of his other contacts. Then the product ρ_jk×ρ_kj measures the combined weight of the contact for j and k together. When this product is large, then j can potentially control the pair relation. More generally, the network control of j is given by

(2)     γ_j = Σ_k=1ⁿ ρ_jk × ρ_kj

For the sake of convenience, let Δ be symmetric, so that each contact is reciprocal. Then the network control of j obtains a simple form, namely γ_j = (Σ_k=1ⁿ ρ_jk) / n_j ⁴. In other words, γ_j is the average of the importance of j for all other actors. Note that the actor j can determine his control γ_j without knowing the whole network. For a symmetrical Δ it suffices when he knows the importance ρ_jk of his own contacts. Thus one finally finds b_j = b_j(γ_j, w, a_j), where n_j is included in γ_j.

Figure 1: Several structures for a network of four

Usually networks with so-called negative ties are studied. A negative tie between a pair j and k exists, when k can close the same transaction with other actors i, when desired. In other words, k can replace (substitute) j by i. It is obvious, that k has more power thanks to the availability of alternatives such as i. In such a situation a large a_j is unfavourable, because then j must spread his power over many transactions⁵. Now a conceivable form of b_j is⁶

(3)     b_j = 1 / (a_j × ln(1 / (w×γ_j)))

Since w×γ_j < 1 holds, the logarithm always is a positive number. This is even an important reason for the introduction of the parameter w. The formula originates from the mathematician and economist K.G. Binmore. The source of your columnist does not elaborate on this functional relation. It does remark, that the theory is applied often in laboratory experiments. Here it turns out that the formula gives a satisfactory empirical description.

It is perhaps clarifying to apply the preceding model to the four networks, which have been described in a previous column. For the sake of completeness, the four networks are presented again, in the figure 1. The networks all have n=4, but the number of contacts varies, namely respectively m=6, 4, 3 and 3. That is to say, in this order w is falling. Let Δ again be symmetrical, so that one has γ_j = (Σ_k=1ⁿ ρ_jk) / n_j. The table 1 contains the matrices R for the four cases, as well as the network control γ_k. Besides b_j is included in the table, under the assumption that a_j=1. In each period each actor closes just one transaction. Due to the formula 2 the differences in γ_j are clearly increased in b_j.

In the all-channel network and in the circle the four actors have the same position, so that they all can exert an equal control. The consequence is that each pair of actors distributes the income π of their mutual transaction equally. Each gets π/2. This changes in the chain, because the actors 2 and 3 in the middle have more control than the actors 1 and 4 at both ends. In their transaction with 1 and 4, the actors 2 and 3 appropriate 66% of π. The wheel sketches a dramatic picture. The actor 1 is like a spider in its web, and can appropriate no less than 90& of π in his transactions with 2, 3 and 4. This is simply due to his favourable position in the network. For, although the figure 1 may suggest otherwise, the actor 1 does not have a social or hierarchical power. The functional equality of the actors follows from the assumption δ_jk=δ_kj.

Laboratory experiments have indeed shown, that the predicted distribution for the wheel in the figure 4 (so with n=4) agrees well with the empirical measurements⁷. However, for the chain a small but yet significant deviation is found. The chain is also studied for n=5, where the result does agree well. It turns out that the formula 3 gives good predictions for a_j=2 and 3, at least for the chain (n=3) and the wheel (n=4). Yet another experiment has studied the wheel, where each spoke consisted of a chain of two actors (in addition to the actor 1 on the axis). According to the result, then the formule 3 overestimates the power of the central actor.

It is obvious that this model is amenable to criticism. It assumes, that all actors are about equally competent. In reality particular personal qualities can evidently lead to a better result. Furthermore, the model is not dynamic. Therefore it can not describe expanding networks⁸. A phenomenon such as power-distance reduction (MAR) is not considered, because the actors can not mobilize and expand their sources of power⁹. In spite of these defects your columnist is very impressed by the performance of the formula 3.

The absence of a hierarchical power is characteristic for experiments, because these commonly study reciprocal groups, where the test persons are brought together just once. Then rules, norms and rol patterns are absent. When a test person has a favourable position in the network (such as actor 1 in the wheel), then he simply represents a talented contact maker ¹⁰. For this reason the formula 3 is also probable for the behaviour on markets, at least in situations without dynamics and the like. Economic actors are often presented as mediators, who are inventive in designing new product. They are skilled in combining the production factors (labour, knowledge, capital, land)¹¹. The best entrepreneurs structure their network in such a manner, that they maintain an optimal control. This gives them a competitive advantage over their competitors¹².

Rent seeking

Often the use of power in the economy takes on the form of rent seeking. Rent seeking is any behaviour, which aims to artificially increase the personal income. In other words, rent seeking leads a a transfer of income without any service in return. It is obvious that rent seeking does require an effort, for instance the lobby in favour of a certain political measure. But that effort does not add productive value, and therefore withdraws labour forces and means from the production. The effort undermines the welfare, and therefore is rejected by economists. A well-known example of rent seeking is the ambition of the enterprises to obtain a monopoly position. For, the enterprise can realize a rent (an extra profit), when it succeeds in eliminating the competition. Thus the income of the consumers is partly transferred to the enterprise, as a rent¹³.

The lobby will generally aim at politics and at the state bodies, because the administration of the state is responsible for the legislation. The administration has the formal power to grant concessions, to establish monopolies, and to protect the industries against competition. However, the industries are not the only seekers of rent. In principle any group can lobby for a rent, including workers, certain categories of households, politicians, and the public bodies themselves. This insight is fairly recent. At the start of the twentieth century, the state was still often depicted as a benevolent dictator, who by nature acts in the interest of the citizens¹⁴. This trust in the state interventions even was the basis for the ideology of socialism, and even more of Leninism. Their failure was perhaps a source of inspiration for theories of powera href="#L15">¹⁵.

Apparently the meaning of rent seeking is rather broad. For instance, even advertising is sometimes considered as a form of rent seeking¹⁶. In this view advertising is simply a lobby. The costs of the lobby can be a significant part of the rent. Sometimes several groups compete with each other for acquiring a rent, such as during the granting of a concession. The chance to gain the rent gives an incentive to make an effort, comparable to the first price in a tournament or lottery. It is even conceivable, that the groups together make more costs than the total rent brings in. Even the entrepreneurial profit could be interpreted as a rent, but that is not the habit. The normal entrepreneurial profit is generally interpreted as a reward for the productive effort of the entrepreneur.

The democracy is a political systen, which somewhat curbs rent seeking. For, the majority of the electorat demands that the political administration tries to further the general interest. As soon as politicians grant favours to particular groups of interest, or to themselves, then they run the risk of losing the following elections¹⁷. In the democracy there is always the option to organize a countervailing power, when the existing order is in danger of degenerating. The hurt citizens can unite in a pression- or interest-group. This view is propagated by the evolutionary institutionalism, which stresses the dynamics and innovation in the society. Nevertheless, the character of this dynamics is still energetically debated among economists.

The economist M. Olson has developed a group theory about the behaviour of interest groups¹⁸. An interest group engages in action in order to extort a rent from the state for her members. This creates a positive external effect, because all persons interested benefit from the rent, without exception. Therefore the persons interested are tempted not to engage in the action by themselves. This is called free riding (in the German language Trittbrettfahren). Their opportunism weakens the group power. It is known from sociology and from the social psychology, that small groups can have a strong cohesion, because the group members have a personal control over each other. One may consult the columns about economic networks and groups. Therefore they can limit the free riding within their group, and optimize their power.

On the other hand, in large groups there is anonymity, which makes them vulnerable to the problem of free riding. Therefore large groups are bad in organizing a countervailing power, as soon as a small group demands a rent for herself¹⁹. In principle the electorat is a countervailing power, but according to Olson it is informed poorly. He predicts that the state will become a plaything of the small interest groups. There is a growth of hurtful institutions, which petrifies the society and stifles innovation. It is remarkable that now Olson propagates, that the state acts as the supervisor of the interest groups. He presents the state as a benevolent dictator, and here ignores, that the state bodies themselves can be rent seekers.

The economist G.S. Becker has developed a model of power, which plays down the conclusions of Olson²⁰. The model of Becker applies a micro-economic approach, where the outcome of individuals is studied. This is called methodological individualism. Suppose that there are two social groups, numbered 1 and 2. The group 1 lobbies for granting a subsidy σ to her members. This must be paid by the group 2, by means of a tax remittance τ. That is to say, one has σ = n×τ, where n is the relative size of the groups 1 and 2. Therefore the group 2 establishes her own interest organization, which lobbies against the measure. The effort of a lobby leads to a power γ_j, and costs c_j, with j=1 or 2. Apparently one has γ_j=γ_j(c_j), with evidently ∂γ_j/∂c_j > 0. Moreover, the rent seeking reduces the social welfare by an amount of Δw = Δw(σ), with ∂Δw/∂σ > 0.

Suppose, that the lobby leads to a continuous change. So one does not have a tournament, with a winner and a loser²¹. Then the variable σ is a function of γ₁ and γ₂. Thus the outcomes for the members of the two lobby-organizations become

(4a)     π₁ = σ(γ₁, γ₂) − c₁(γ₁) − Δw(σ(γ₁, γ₂))
(4b)     π₂ = - (1/n) × σ(γ₁, γ₂) − c₂(γ₂) − Δw(σ(γ₁, γ₂))

Note that for both lobby-organizations π_j depends on the lobby of the other party. The group j does not know the strategy of the opponent, and can not influence it. For, the lobby is aimed at the state. The optimization of π_j requires ∂π_j/∂γ_j = 0, where this equation must be formulated for each possible γ_k of the opponent. The result is

(5a)     ∂σ/∂γ₁ = ∂c₁/∂γ₁ + ∂Δw/∂γ₁
(5b)     ∂σ/∂γ₂ = -n × ∂c₂/∂γ₂ − n × ∂Δw/∂γ₂

The set 5a-b cab be used for calcultating the optima γ_o,1 and γ_o,2. For, the functions σ and Δw are known, and moreover each organization knows her own γ_j. The expressions γ_o,1(γ₂) and γ_o,2(γ₁) are called the best-reaction functions of respectively the organization 1 and 2. The reactions are called Cournot-Nash behaviour. The optimization implies that each lobby-organization can increase her power, until the increase of the outcome π_j becomes less than the increase of the costs c_j. Here the two organizations run up each other, because their power has an opposite effect on σ. In other words, one has ∂γ₂/∂γ₁ >0 and ∂γ₁/∂γ₂ >0. Finally this ends in an equilibrium γ_o,1(γ_o,2), namely the intersection of the two best-reaction curves.

A concrete example can clarify this²². Let σ = 10×γ₁ − 5×γ₂, and Δw = σ²/1000. That is to say, the welfare decreases in an exponential way, according as the subsidy (rent) increases, Therefore even the group 1 will not want to raise σ without limits. Moreover, Becker assumes, just like Olson, that the lobby for the subsidy is started by a relatively small group 1. The counter-movement 2 is larger, and therefore less cohesive. This has the consequence, that one has ∂γ₁/∂c₁ > ∂γ₂/∂c₂. Therefore assume that one has γ₁ = √(c₁) / 20, and γ₂ = (c₂)^1/3 / 20. Letn=1, so that σ=τ. A calculation with the set 5a-b yields the best-reaction functions γ_o,1 = ¹/₃×γ₂ + ¹⁰⁰/₃ and 3×γ_o,2² + γ_o,2 = 2×γ₁ + 100. The intersection of these two curves is (35.8, 7.4). The figure 2 shows the two best-reaction curves.

Figure 2: Best-reaction curves of
    power and countervailing power

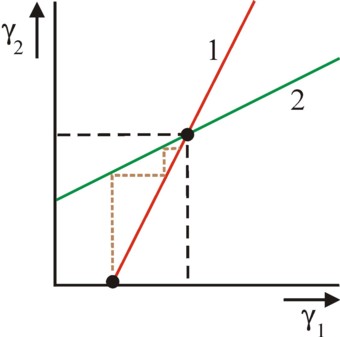
Apparently the lobby of the group 2 is useful. For, without the lobby of the group 2 (c₂=0) the group 1 realizes a rent σ = 333 thanks to her effort with a size c₁ = 55.6, and a profit π₁ = 167. In this situation the group 2 makes a loss of π₁ = -444. In the equilibrium the rent of the group q is merely σ = 321, despite the increased costs c₁ = 64.1. The profit is decreased to π₁ = 154. Now the group 2 must naturally make costs, namely c₂ = 20.2. But thanks to the decrease of σ the group 2 yet keeps her loss constant at -444. For completeness, your columnist notes, that this example does illustrate the usefulness of the countervailing power, but also is constructed in a rather artificial manner²³.

Becker interprets the result of his model in the following way. In society various interest groups compete with each other. The mutual competition prevents that a single group can enrich herself unjustly at the cost of the rest. Especially large groups will often defend the general interest, or at least take it into account. Moreover, the large groups can engage in transferring information to the voters. These groups use the public opinion to exert pressure on the politicians to reject unfair demands of small pressure organizations. This also curbs the welfare loss Δw. All in all, Becker is positive about the lobby organizations, because they also draw the attention to the existing injustices, and by means of their call for institutional innovation improve society. Note furthermore, that this positive judgement can not be derived from his formal model.

Furthermore, criticism on the view of Olson is warranted because of his neglect of neutral organizations²⁴. Think about the scientific institutes, the independent media, and other independent inspectors. A wise state will educate its citizens well, so that they are capable of evaluating the lobby of the various interest groups. In such a society, lobby organizations give beneficial signals about the diversity of the existing needs. The loyal reader may remember, that according to the sociologists J.S. Coleman and R. Putnam the interest groups can even be identified as a social capital. Thanks to the social capital the economic transaction-costs are reduced.

Worth mentioning is the view of the mentioned Frijters on rent seeking²⁵. His taxonomy makes an explicit distinction between small and large circles, precisely because of the difference in cohesion. He states that the class-theory can be interpreted as a theory of rent seeking, especially in the version of Olson. A striking hallmark is the emphasis on material interests. Frijters objects, that group processes are influenced by the cohesion and by the internal morals of the group. Besides, the theory of rent seeking pays little attention to the methods, that are used to exert power and influence. All in all, Frijters is positive about the lobby, and his views are congenial to those of Coleman and Putnam. By the way, note that Frijters is quite critical about the lobby of the banana industry in Australia.

Note that the theory of rent seeking is supported by the social psychology. People join a group or a circle in the expectation, that the membership yields material and psychological benefits²⁶. The group has a common goal, and together gains power. The group reinforces the self-esteem. Furthermore, groups tend to polarize, which can result in the pursuit of high incomes. The members have a favourable opinion about their own group (ingroup favouritism, self-serving bias)²⁷. The psychology states, that the competition for rare resources leads to conflicts between groups. This creates a hostile attitude, which is expressed in prejudices and discrimination. The group will become frustrated by the organized countervailing power. Therefore it is important, that institutions exist for solving the clashes between the groups in a peaceful manner²⁸.

Your columnist can not refrain from speculating about the meaning of rent seeking for the trade union. For, she is an eminent example of the ambiguous character of the lobby. The trade union movement wants to redistribute the incomes. During the nineteenth century, the heydays of classical liberalism, the emerging trade union movement is the exponent of the justified needs, which live in the proletariat. According to marxism, the entrepreneurs appropriate an excessively large part of the produced surplus value. The socialist trade unions want to solve this radically by expropriating the property of the capitalists. The collective agreement monopolizes the formulation of the labour contract, but is nonetheless valued as a useful institution. The minimum wage and the protection against dismissal are legal forms of rent seeking, which are of old controversial.

During the sixties the trade union movement gains power thanks to the full employment. It demands high wages to such an extent, that the profitability of the industries is structurally affected. This is truly a weird period, because under the influence of the New Left the media and even parts of science give up their neutrality, certainly in the Netherlands. Perhaps Olson mainly refers to this spirit of the time in his model. Then the trade unions demand a (too) radical participation in management. The social trade union movement emerges, so that unions become quasi-political bodies. During this period the rent seeking had dogmatic and irrational traits. Nowadays the trade union movement struggles to prove its reason of existence - incidentally just like many other collective associations. Paid propagandists enter the enterprises as organizers.

The tournament

The theory of the principal-agent shows, that workers can seek rent. This problem occurs for instance in shift work, where the labour productivities ap_j of the separate workers j can not be distinguished. That is to say, an individual reward, based on result, is not possible. Now the enterprise can decide to base the individual wage on a tournament-system²⁹. Here the extra efforts e_j are registered for each worker j, and that e_j determines the individual wage. Since the effort does not have a direct relation with productivity, the wage height is rather arbitrary. In the tournament system the wage has the form of a "first price". Suppose that the workers are risk-neutral, such as in the case of managers, as far as they possess some personal capital. Their ap_j can actually not be measured, and the tournament appeals to their already competitive attitude.

The present model considers a tournament between two workers j=1 and 2. The standard wage is π, and the first price is a bonus or premium Δπ on top of that wage. Let e_j be the truly supplied effort by j. It is accompanied by costs c(e_j). Suppose that the enterprise can register e_j only inaccurately, so that it measures a value η_j = e_j + ε_j. The uncertainty ε_j in the registration is represented by the density function f(ε_j), which for the sake of convenience is assumed to be the uniform distribution (f=1), on the interval [-½, ½]. Let p(e_j) be the probability, that j wins the premium Δπ with his effort. Thus the expected utility of j becomes

(6)     Eu_j(e_j, Δπ) = p(e_j)×Δπ − c(e_j)

Figure 3: Density functions f(ε) and g(Δε) and distribution function G(Δε)

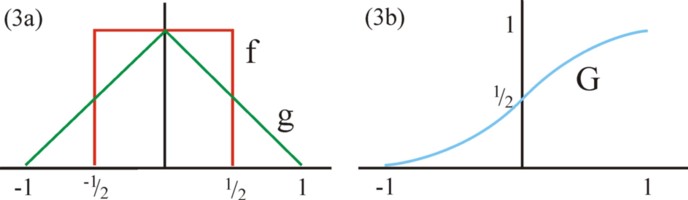
Suppose for the sake of convenience, that one has c(e_j) = β×e_j². The worker j maximizes his utility by means of Δπ × ∂p/∂e_j = 2×β×e_j. The theoretical challenge is to calculate ∂p/∂e_j. The worker 1 wins the tournament, when η₁>η₂ holds. This is identical to e₁ − e₂ > ε₁ − ε₂ = Δε. Since Δε is at most 1, the winner is clear for the case |e₁ − e₂| > 1. When this does not hold, then a somewhat more complex argument is needed. Since the probability distributions f(ε_j) are known, the density function g(Δε) can also be determined with the help of statistical theory. It is an equilateral triangle on the interval [-1, 1], as is shown in the figure 3a ³⁰. The corresponding cumulative distribution function G(Δε) is shown in the figure 3b. Note that the probability p satisfies p(η₁>η₂) = p(Δε < e₁ − e₂) = G(e₁ − e₂). Therefore one has ∂p/∂e_j = g(e₁ − e₂). So in the optimization of Eu₁(e₁, Δπ) the two "legs" of the triangle in the figure 3 b must be distinguished

(6a)     e_o,1 = (1 − (e_o,1 − e₂)) × Δπ / (2×β)     for e_o,1 > e₂
(6b)     e_o,1 = (1 + e_o,1 − e₂) × Δπ / (2×β)     for e_o,1 < e₂

These are the situations of uncertainty, which lie in the area |e₁ − e₂| ≤ 1. The figure 3b shows, that the worker can increase his probability p by making a larger effort. He wil optimize Eu₁ by equating e_o,1 to (1 + e₂) / (1 + 2×β/Δπ) and (1 − e₂) / (-1 + 2×β/Δπ) for respectively the cases 6a and 6b. This is the best-reaction function for worker 1, and the one for worker 2 is naturally similar. See the figure 4. Suppose that initially e_j=0 for both workers. The competition between the two workers mutually motivates them to outdo each others effort, in conformity with their best reaction, until this no longer pays. Again there is a Cournot equilibrium, which is located in the intersection of both best-reaction curves. In the equilibrium one has e_o,1 = e_o,2 = Δπ / (2×β), so that thanks to the tournament a total extra effort of Δπ/β is made.

Figure 4: Best-reaction curves of
    efforts in tournament

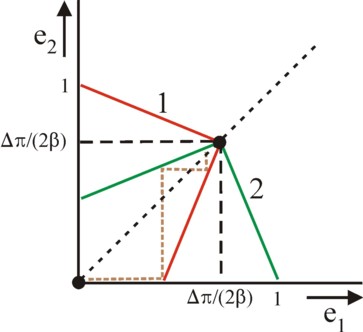
The tournament could be called a rat race³¹. The tournament stimulates both workers to make an extra effort. Since they end in the equilibrium e_o,1 = e_o,2, it would have been better to remain in the point (0, 0). However, this point is unstable, because the workers do not know each other's reactions. One could complain, that the tournament raises the work load. And the material incentive can lead to the suffocation of the intrinsic motivation (crowding out). On the other hand, the extra effort has a positive effect on the welfare. And this is beneficial for everybody. The culture of the enterprise must be such, that the personnel can act decently in the tournament³². For, the tournament is actually a struggle for power, where it pays to belittle and slander the effort of the other. The cooperation can become impossible, when cohesive group morals are not stimulated at the same time.

So it does not surprise, that the social psychology is ambiguous about competition between group members. The psychology acknowledges that incentives are needed in order to stimulate the workers to make an effort³³. But they cause various side effects. Competition can seduce the workers into stretching their competences³⁴. This can even lead to conflicts about group targets³⁵. Prejudices and hostility can emerge within the group. The participants in the tournament can each form their own coalition within the enterprise³⁶. This conflicts with the idea behind the enterprise, which is precisely established in order to further cooperation.

The formation of coalitions can be a conscious choice of the enterprise, such that it includes self-steering teams within its organization structure. In the former Leninist states the economy was permeated with a "socialist" idea of competition³⁷. Then the competition is seen as a hallmark of the healthy personality, provided that it is embedded within humanist relations. The enterprises are encouraged to exceed the planned task. Working groups (collectives, brigades) are formed in the workshop³⁸. The individual wage is supplemented with premiums and collective rewards, which are coupled to the performance of the working group³⁹. The premiums are roughly 10% of the wage. Although the premiums are primarily coupled to productivity, they are also paid for innovations (Neuerer-Bewegung). The premiums are also meant to incite the weak groups (tournament effect).

1. In Eastern Europa, the Soviet-Union, China and parts of South-America, marxism has been a state doctrine for a long time. After the Second Worldwar, Western-Europe developed the neo-marxism, an adapted version which recognizes the autonomous role of the state. Worth mentioning are N. Poulantzas, R. Miliband (the father of the well-known New Labour politicians Ed and David), and L. Althusser. Your columnist definitely wants to read their works, once. This current still has some supporters, but your columnist believes it is not useful. (back)
2. See chapter 8 in Rational-Choice-Theorie (2011, Juventa Verlag) by N. Braun and T. Gautschi, notably the paragraph 8.2. The loyal reader recognizes this approach from the previous columns about bargaining about collective agreements. The approach of Nash has naturally already been applied before. For instance, Sam de Wolff describes on p.366 and further in Het economisch getij (1929, J. Emmering), and the annexes on p.449, the bargaining about a collective agreement, where the workers optimize their utility on the value κ, and the entrepreneur maximizes his profit.
   A special case of this type of problems is discussed on p.129 and further in Group dynamics (1990, Brooks/Cole Publishing Company) by D.R. Forsyth. Here it merely concerns communication networks, where the transaction consists of a transfer of information. Then the challenge is not the distribution of income, but an effective deliberation in order to quickly execute an order or task. Incidentally, information also has a value, because persons feel neglected, when they are not informed. Moreover, a lack of information impedes the concerned person in his actions, and thus in his unfolding. (back)
3. When your columnist understands it well, then a_j transactions are divided over the n_j contacts. That is to say, the number a_j refers to the period, and not to the separate contact k. See p.223 in Rational-Choice-Theorie. The same theme is discussed on p.116 in De kern van de sociale psychologie (1990, Van Loghum Slaterus) by P. Veen and H.A.M Wilke. Your columnist read this book already 22 years ago. (back)
4. Due to the symmetry of Δ one has Σ_i=1ⁿ δ_ik = n_k. It follows that ρ_jk = δ_jk / n_k. Insertion gives γ_j = Σ_k=1ⁿ δ_jk × δ_kj / (n_k × n_j) = (Σ_k=1ⁿ δ_jk² / n_k) / n_j. Since δ_jk has a value of 0 or 1, one has δ_jk² = δ_jk. This implies γ_j = (Σ_k=1ⁿ δ_jk / n_k) / n_j = (Σ_k=1ⁿ ρ_jk) / n_j, which was to be proved. (back)
5. On p.112 and further in De kern van de sociale psychologie a distinction is made between three factors of power: the value π of the transaction, the availability of alternative offers for the same transaction, and the number of transactions, that j wants to conclude with k. This book stresses, that the power of k originates from the dependence of j. (back)
6. In paragraph 8.2 of Rational-Choice-Theorie the situation of the positively connected network is also considered. The hallmark of a positive connection is, that the transaction is complementary. That is to say, the actor k wants to execute coupled transactions, wherein he wants to acquire various goods, which all belong together. For instance, when one buys a personal computer, there is also a need for a monitor, a mouse and a keyboard. It turns out that in the positively connected network b_j = a_j / ln(1 /(1 − w×γ_j)) is a useful assumption. Your columnist will not elaborate this case. (back)
7. See p.231 in Rational-Choice-Theorie. For the other experimental results see p.232, p.234 and p.235. On p.116 in De kern van de sociale psychologie a similar experiment is described. (back)
8. These deficits are mentioned on p.235-236 in Rational-Choice-Theorie. Your columnist concludes that in the experiments the value π was apparently always equal for all pairs of actors. This raises the question what will happen when π differs for each pair. It seems logical, that actors first want to conclude the transactions with the largest value. Then transactions with a relatively large π contribute to the power of the concerned actor. Rational-Choice-Theorie does not elaborate on this point, but p.113 of De kern van de sociale psychologie stresses it. (back)
9. See p.109 and further in De kern van de sociale psychologie. According to this book, influence originates from a mobilized power. See p.42 and p.114 there for the MAR. (back)
10. The irrelevance of the hierarchy also becomes apparent in the chain. For, it can be interpreted as a completely vertical organization. Nevertheless, the table 1 shows, that the actor "at the top" has less power than the actors in the middle layer. (back)
11. In chapter 3 of The economics of business enterprise (2002, Edward Elgar Publishing, Inc.) by M. Ricketts several stereotypes of entrepreneurs are discussed. For the present theme, two stereotypes are of particular importance. According to I. Kirzner an entrepreneur is someone, who sees opportunities to add value. It is simply a creative individual with a nose for money. According to J.A. Schumpeter the entrepreneur is someone, who engages in innovative break-throughs. This stereotype is a revolutionary, much more than according to Kirzner, who radically changes the economy and even the society. In this way Schumpeter even tries to explain the economic conjuncture. (back)
12. On p.132-133 in Group dynamics it is remarked, that the central position in a network is often accompanied by a high status. This high states can become a factor of power in itself, because the other actors have high expectations about the central entrepreneur. In Group dynamics this statement concerns hierarchies. However, it can be expected, that in market-like relations the financial motives will dominate over the social ones (status, mutual obligations, social capital and the like). There is a strong incentive to compete. (back)
13. This introductory argument is based on p.199-203 in The economics of business enterprise. The economists G. Tullock and J.N. Buchanan have made an important contribution to this theory of power. A more succinct argument can be found on p.334-339 in Political economy in macroeconomics (2000, Princeton paperbacks) by A. Drazen. (back)
14. See p.357 in Neue Institutionen-ökonomik (2007, Schäffer-Poeschel Verlag) by M. Erlei, M. Leschke and D. Sauerland. (back)
15. Your columnist possesses a fairly larg collection of books about Leninist economics, and has really studied them. Until far in the twentieth century Leninism has propagated the social dominance of the state. Then the state is again subjected to the Leninist party, itself a political monopoly. On paper the Leninist system makes sense, but despite many attempts the results remained unsatisfactory. In the Gazette five columns about this theme deserve a special mention. They discuss: (a) several popular-scientific books from the GDR, (b) a study of the Russian R.A. Beloussov about groups interests and planning, (c) a study of the Russian K.K. Val'tuck about social needs, (d) a study of the German R. Stollberg about the Leninist labour relations, and (e) finally an analysis of the failure of the planning system. Since then, your columnist has somewhat lost his enthusiasm. Amateurs can also consult the ideas of the social-democracy in the Gazette, here and there. (back)
16. See p.37 and p.54 in Industrial organization in context (2010, Oxford University Press) by S. Martin. Of old, the socialists believe that the costs of advertising are a social waste. The socialization-report of the SDAP refers to these costs as an argument in favour of socialization, and later in favour of the public branch organizations. The Leninist system used commercial advertising albeit on a moderate scale. The political advertising for the Leninist party dominated the whole social life. Here the preferences of the population were neglected. (back)
17. This is explained clearly in paragraph 6.2 of Neue Institutionen-ökonomik. (back)
18. Here paragraph 6.2.4.1 in Neue Institutionen-ökonomik is consulted. (back)
19. Those who want can model this with game theory, as the problem of the prisoner's dilemma. In a large group cooperation is rare. In a small group the matrix of outcomes changes, because the group members discipline each other by means of rewards and punishments. (back)
20. See paragraph 6.2.4.2 of Neue Institutionen-ökonomik. (back)
21. On p.339-342 of Political economy in macroeconomics Drazen describes a model of a tournament, where two lobby organizations compete for the acquistion of the same concession. This model is mainly interesting, because it illustrates the application of the mixed strategy. In a pure strategy the two groups would continue to endlessly bid for the concession. An equilibrium is only possible, when they choose a mixed strategy, where both run the risk of losing the concession. In such a situation the groups have an expected profit of Eπ_j, with a height that depends on the probability, that they are willing to make costs c_j. Drazen shows, that at most two groups will participate in such a tournament. Your columnist does not present this model, because its practical meaning is unclear. (back)
22. This example is copied from p.392-395 van Neue Institutionen-ökonomik. (back)
23. Your columnist has spent a day for finding a simpler example. Notably the cost functions seem excessively complicated. In Neue Institutionen-ökonomik these are chosen, because according to p.390 the authors want to satisfy the condition ∂²c_j / ∂γ_j² > 0 for j=1 and 2. That is to say, the marginal costs ∂c_j/∂γ_j exhibit a rising trend with γ_j for both organizations. This is indeed logical, but it makes the calculation unpleasantly complex. The situation would be much simpler with γ₁ = c₁ and γ₂ = √(c₂). It is true that this implies constant marginal costs for the group 1, but one still has ∂γ₁/∂c₁ > ∂γ₂/∂c₂, at least for γ₂ > ½. Your columnist has used this to construct his own example. Let σ = 5×γ₁ − γ₂, and Δw = σ². Suppose that n=2, so that always 2 members of group 2 must remit for the subsidy to each member of group 1. The set 5a-b yields the best-reaction functions γ_o,1 = ¹/₅×γ₂ + ²/₂₅ and γ_o,2 = ⁵/₂×γ₁ + ¹/₈. The intersection of these two curves is (²¹/₁₀₀, ¹³/₂₀). This example looks elegant, but it turns out that it has confusting consequences, which are conveniently evaded in the example of the book. Namely, due to the formula 5a σ constantly has the value 0.4 in this example. That is to say, as soon as the group 1 tries to increase σ, the group 2 will immediately compensate this attempt. However, the group 2 never succeeds in decreasing σ in a durable way. Therefore the loss π₂ of the group 2 increases from -0.36 at γ₂=0 to -0.783 at the eqilibrium γ₂ = ¹³/₂₀. At the same time the profit π₁ of the group 1 decreases from 0.16 to 0.03. Apparently everybody is worse off by the countervailing power (unless the group 1 would end her lobby because of the falling profit). One wonders, why nevertheless the group 2 yet chooses to lobby. The reason is that the group 2 is not able to foresee the reaction of the group 1. And on the dynamic path on the way to the equilibrium the group 2 again and again has temporary successes. For example, the best-reaction curve of group 2 in the situation with c₂=0 shows, that now it must develop a power γ₂=0.245. That temporarily reduces σ to 0.155, and π₂ to -0.162. Unfortunately, the reaction of the lobby-organization 1 will soon follow. All these observations show, that the example in the book is not a standard situation. (back)
24. See p.398 in Neue Institutionen-ökonomik. (back)
25. See p.215-217 in An economic theory of greed, love, groups and networks (2013, Cambridge University Press) by P. Frijters and G. Foster. (back)
26. See paragraph 9.1.2 in Sozialpsychologie (2008, Springer-Verlag) by L. Werth and J. Mayer, and chapter 3 in Group dynamics. (back)
27. See paragraph 9.3.2 in Sozialpsychologie and p.308 in Group dynamics for the polarization, and p.408 in Sozialpsychologie for ingroup favouritism. See chapter 9 in An introduction to behavioral economics (2008, Palgrave Macmillan) by N. Wilkinson for the self-serving bias. (back)
28. See paragraph 10.3.2 in Sozialpsychologie. Prejudices are a greeding ground for emotional actions. But on p.441 it is remarked, that aggression is sometimes used instrumentally in order to realize the personal goals. See paragraph 11.2 in Sozialpsychologie and p.368 in Group dynamics for frustration as a source of aggression. The inclination of reciprocity can be negative, and end in retribution (p.369 in Group dynamics). (back)
29. The contents of the present paragraph is based on paragraph 6.6 in The economics of business enterprise, as well as the annex on p.214-216. The model has some resemblance with the one of Drazen on p.339-342 of Political economy in macroeconomics. However, there the uncertainty results from the application of a mixed strategy, and not from an imprecise registration. (back)
30. One may consult any book about statistics. Your columnist still owns from his physics study Introduction to mathematical statistics (1978, Macmillan Publishing Company, Inc.) by R.V. Hogg and A.T. Craig. There on p.40 the explanation is: let z = x − y, where x and y have a uniform distribution f=1. For z in [-1,0] one has p(x-y < z) = ∫_-z¹ ∫₀^ζ+z f(ξ) × f(ζ) dξ dζ = ∫_-z¹ (ζ + z) dζ = ½ + z + ½×z². This is the cumulative distribution function G(z) van x-y. It results in the density function g(z) = ∂G/∂z = 1+z. This is the left side of the triangle. For z in [0,1] one has p(x-y < z) = 1 − p(x-y ≥ z) = 1 − ∫_z¹ ∫₀^ξ-z f(ξ) × f(ζ) dζ dξ = 1 − ∫_z¹ (ξ − z) dξ = ½ + z − ½×z². It results in the density function g(z) = ∂G/∂z = 1-z. This is the right side of the triangle. Those who are visually oriented, may appreciate the text about market power. The calculated integrals yield the area of the "yellow" and "orange" planes in this text. However, the boundary between the yellow and orange planes is from the upper left to the lower right, whereas the line y = x − z is from the lower left to the upper right. (back)
31. See p.193 in The economics of business enterprise. (back)
32. See p.195 in The economics of business enterprise. (back)
33. The psychological term for the inclination to laze and be idle in group tasks is socal loafing (p.269-274 in Group dynamics, paragraph 9.2.1 in Sozialpsychologie). The solution is found in evaluation, trust, and responsibility. (back)
34. See p.115 and further in Group dynamics. (back)
35. Forsyth dscribes on p.353 and p.359 in Group dynamics the conflict between Sculley and Jobs about the goals of the computer supplier Apple. (back)
36. See chapter 12 in Group dynamics for the discussion of these problems. (back)
37. See paragraph 2.3.3 in Soziologie der Arbeit (1988, Verlag Die Wirtschaft) by R. Stollberg. (back)
38. See paragraph 2.3.5 in Soziologie der Arbeit. (back)
39. See paragraph 6.2 (notably p.173) in Einführung in de politische Ökonomie des Sozialismus (1974, Dietz Verlag), edited by W. Becker, G. Schulz and K.-H. Stiemerling. (back)