The saturation in indifference curves

First insertion on Heterodox Gazette Sam de Wolff: 11 july 2013

E.A. Bakkum is a blogger for the Sociaal Consultatiekantoor. He loves to reflect on the labour movement.

The common neoclassical paradigm is by necessity limited to a specific behaviour of consumers. When it is accepted that utility is measurable and can be saturated, then it becomes possible to study the satisfaction of needs in a more general sense. This column starts with the analysis of the utility slope. Next a model of indifference curves by the Russian economist K.K. Val'tukh is explained. His formula can be applied in practice for the description of the target function in planning calculations with a many-period optimization by means of an intertwined balance.


Various types of utility functions

There are utility distributions of all sorts. In the previous column three types of utility distributions have already been presented:


Once more cardinality for utility functions

In a previous column it has been explained, how originally the paradigm of the marginal utility was built on the assumption, that utility can be measured. When the idea of a utility measure is abandoned, and an ordinal utility is preferred, or even the concept of utility is entirely avoided, then also the intuitive appeal of this consumer theory disappears. Moreover her applicability is then limited to certain special situations. These are convincing arguments in favour of the measurable utility (cardinality).

Figure of utility functions
Figure 1: Utility functions with saturation

At this point it is worth mentioning, that the assumption of a measurable utility is essential for the study of consumer preferences in situations with uncertainty. The attention of your columnist was drawn to this phenomenen by the excellent economics weblog of Robert Vienneau. A simple example illustrates the idea of these studies3. Suppose that the acquisition of a product unit has a utility u1, and the acquisition of two product units has a utility u2. Now ask the individual to choose between: (a) obtain one product unit with certainty, or (b) obtain two product units with a chance p, where thus a chance 1 − p exists of getting nothing.

Now it is intuitively clear, and it can be shown, that both options will be equivalent for him in the situation, where one has p = u1/u2. The rational individual must be able to estimate his own p, and thanks to this comparison can apparently also scale both utilities. Then he also knows their relative difference Δu/u2 = 1 − p. Just like in the previous column about cardinality, which was just mentioned, the utility can be measured simply by asking the individual!

Thanks to the assumption of cardinality it is fairly simply to construct a model, where the acquisition of a product has become a burden. The figure 1 illustrates how in the beginning the utility u(x) rises with the quantity x of the product. At the point x=χ the saturation occurs, and henceforth more of the product will diminish some of that utility. The present-day common neoclassical paradigm of the marginal utility can actually not cope with this case, and that his surprising consequences.

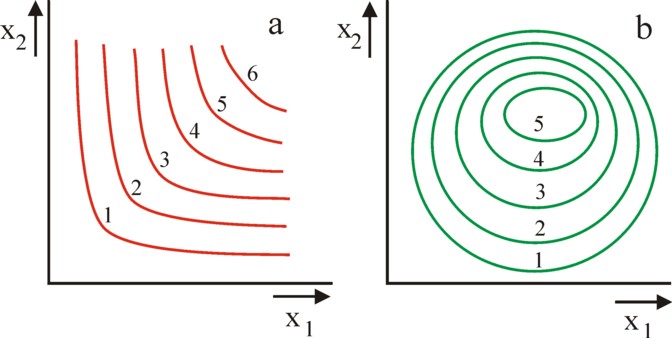
Figure of iso-utility curves
Figure 2: Indifference map without saturation (a) and with (b)

Namely, consider first the behaviour of the utility function according to the common neoclassical view. In its simplest form this paradigm portrays the utility by means of the wellknown iso-utility or indifference curves. They are purely individual. Each curve connects product combinations with an equal level of utility. According as the quantity x of products increases, and the distance from the origin becomes larger, there is an obvious rise of the utility. The figure 2a gives an illustration of such a field or map of curves. The individual will exchange products (or actually their properties) with other individuals just long enough to reach the maximum attainable indifference curve for his situation. The indifference map is a wall, as it were, which is climbed,

The problem of the figure 2a is, that the utility continues to grow. For it is simply not true, that someone is better off with for instance 100 watches instead of 99 (unless he is a collector). They become a nuisance and a burden4. A burden has a negative (marginal) utility, and will decrease the total utility of the individual. The figure 1 illustrates this for the one-dimensional case. Thus in the two-dimensional case the utility wall changes into a utility peak. This is displayed in the figure 2b. In the common introductory text books this aspect is often ignored, but not always. See for instance in Micro-economie by Dietz, Heijman and Kroese5. It is evident that the perspective on the whole concept of utility changes, as soon as the falling utility is taken into account6.

In the previous column about cardinality it is stated, that an individual can never know the entire utility field, such as is displayed in the figure 2. That is impossible for humans. It has been explained how according to the Russian plan-economist K.K. Val'tukh and the Dutch economist Bernard van Praag the individual disposes of a limited and changing set of preferences. Suppose that at a certain time t the possessions in his situation consist of x(t), then he merely knows with some accuracy the indifference curves in the immediate vicinity of that point. According as the distance from that point increases, his indifferences become more vague. He has simply never considered them. Moreover the curves are continuously affected, because new products appear on the markets, which require the acquisition of experiences.


A practical application of indifference curves

There are several reasons for paying attention to the Leninist vision on the utility function. Thanks to the ideological controversy between the capitalist and planned economies the economists from the former Leninist states do not suffer from the stifling encapsulation of the neoclassical paradigm of marginal utility7. And their efforts are directed towards practical applications, more than in the west. The value-free and fundamental research consisted mainly of ideological policy studies and criticism of the capitalist system. It focused on the international "methodological debate". A disadvantage of this ideological approach is that the paradigm of marginal utility was distrusted. In fact the Leninist science seems to be more interested in the debate about the social policy than in formal-analytical arguments.

So although the Leninist planned economists in general did not analyze the "subjective" utilities, this kind of research was not prohibited. Your columnist disposes of the book Entwicklungs-proportionen und Befriedigung der Bedürfnisse by the Russian economist K.K. Val'tukh, which contains many original ideas8. This paragraph gives a bird's-eye view of some of them. His model of the indifference curves has the unique hallmark, perhaps by necessity in order to obtain funding, that it has really been used in practical computations.

Val'tukh develops a theory for the social welfare function U(x1, ... , xN), such as is described just now in the third point of the first paragraph. The utility function U has indifference curves, just like any other, but in this case they refer to the central planning agency. This agency is influenced by the opinions of the government, the ministries, the industrial and trading organizations, science, etcetera. Val'tukh imagines a utility shape, similar to the one in the figure 1. Here he stresses, that in fact the utility does not refer to a single product, but merely to a situation with a total package of goods. The separate products obtain their utility in the historical and technological context of the collectieve wealth and richess.

Val'tukh places the concept of utility completely within the framework of the planning process, his employer. At a certain time t=0 the planned production has a given level, which is called by Val'tukh the normal level. Thanks to the planning process the productivity grows in a durable manner, so that a drop below the normal level will never occur. The planning process is only interested in the future growth above the normal level. So whenever this paragraph will discuss quantities x, this refers only to the growth above the normal level. The growth can be planned, and this is laid down in the so-called perspective plan. The corresponding time horizon covers a period of five or more years.

The planning agency has the mission to satisfy the needs of all citizens in an optimal way, within the boundary of the perspective plan. According to Val'tukh the satisfaction of needs is simply the supply of certain services, which in the eyes of the planning agency are desired by the citizens. Needs are reasonable and their satisfaction is necessary in the given historical situation. The level of the perspectivistic needs is derived from the situation, where the demand is exactly saturated. In other words, the perspectivistic level is x=χ. A higher level can not occur, because that is undesirable, and therefore forbidden by the plan.

Thus the planning agency must find a level of needs, which satisfies the condition 0 ≤ xn ≤ χn for all n. In the search process the quantities xn are adapted until finally u(x ) is maximal. Note also, that on a difference curve U = constant the exchange rate or marginal substitution rate ∂xj/∂xk must be negative. When a unit of k is given up, then the lost utility must be compensated by additional units of j. Therefore the convex side of the indifference curve points towards the axes of the field.

In an indifference field (x1, x2) the rise of xj (j=1,2) above χj can only be prevented by imposing limitations on the exchange rate ∂xj/∂xk. As soon as saturation occurs for one product, then on the curve a further decrease of a quantity of the other product can be impeded by demanding an infinite quantity of the saturated product as a compensation. This requirement can naturally never be met. In order to be precise: when one has x1=χ1, then the exchange rate ∂x2/∂x1 must be zero. Here the indifference curve is horizontal. And when one has x2=χ2, then the exchange rate ∂x2/∂x1 must be infinite and negative. Here the indifference curve is vertical.

In the same way the curve must never fall below the coordinate axes x1=0 en x2=0. For that would lower the normal level. So at the intersection of the indifference curve and the xj axis the increase of xj must not be compensated by a further decrease (towards negative values) of xk. That is to say, when one has xk=0, then the exchange rate must satisfy ∂xj/∂xk=0. Thus xj can only increase with an additional unit, when in compensation xk decreases with an infinite number of units, which is obviously impossible. This means in fact, that a difference curve ends, as soon as she intersects the axis.

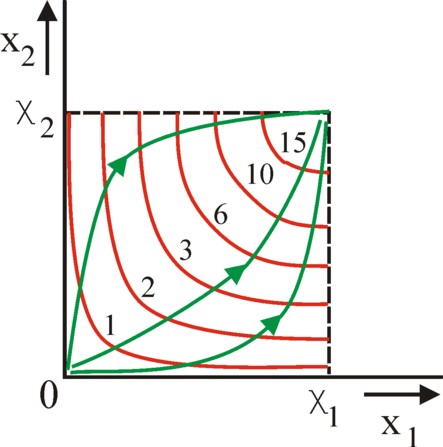
Figure of iso-utility curves
Figure 3: Iso-utility curves according to Val'tukh
with saturation and three paths of growth

The figure 3 displays the indifference field of the planning agency, in line with the model of Val'tukh. Eventually the planning agency wants to reach the point x=χ, which is the top of the utility mountain, similar to the one in the figure 2b. The planning agency must choose a growth path towards the top, for instance one of the four alternatives in the figure 3. Since the utility function u will behave differently on each growth path, the optimal alternative must be selected. It is obvious that the growth path is a function of the time t.

Note that the determination of the growth path is fundamentally different from the determination of the "growth path" for individuals, in the manner of the neoclassical paradigm of the marginal utility. For the growth path of an individual is determined by the personal income. But the planning agency has the power to manage also the supply of the products. Here the production capacity must be taken into account, which is available at the time t=0! The management of the supply (in casu the production) implies, that the planning agency can vary the value (the cost price) of the products.

For the practical application of the indifference field it is convenient to write it in a mathematical form. Therefore Val'tukh tries to find a formula for the exchange rate ∂x2/∂x1. In any case this formula must satisfy the boundary condition ∂x2/∂x1=0 in x1=χ1 and in x2=0, as well as the boundary condition ∂x2/∂x1 = infinitely negative in x2=χ2 and in x1=0. The simplest formula, which satisfies these conditions, is

(1)     ∂xj/∂xk = - (xj / xk) × (1 − xk/χk) / (1 − xj/χj)

The formula 1 can be solved with the method of the separation of variables. This leads to

(2)     (1 − xj/χj) × (1 / xj) × dxj = (xk/χk − 1) × (1 / xk) × dxk

The left-hand side and the right-hand side can both be integrated easily. The result is

(3)     ln(xj) − xj / χj = xk / χk − ln(xk) + c

In the formula 3 ln(.) is the function of the natural logarithm, and c is an arbitrary integration constant. When both sides are inserted in the argument of the e-power e(.) or exp(.), then one finds the relation xj × xk / exp(xj/χj + xk/χk) = constant. In the more general form with N products the indifference curve changes into a hyperplane, which is defined by the formula

(4)     (Πn=1N xn) / exp(Σ n=1N xn/χn) = C

In the formula 4 Π is the symbol for the multiplication of the variables, and Σ is the summation symbol. On the indifference curve the quantity C is a constant. Now vary xj, and keep the other xk with k≠j constant. Then one has (not stated in the book of Val'tukh)

(5)     ∂C(xj)/∂xj = C(xj) × (χj − xj) / (χj × xj)

The formula 5 proves, that C is a rising function of xj. Therefore C can be interpreted as a quantity, which indicates the utility of the package (x1, ..., xN) of goods. In other words, C plays the role of the utility value on the indifference curve. Thus U(x1, ..., xN) = C can indeed be interpreted as the equation of an indifference plane. As far as your columnist can see, this utility function is in principle an ordinal one. The formula 4 is very convenient for a planning agency, that searches for an optimal arrangement of the economic system. For the formula makes it possible to mutually compare in a qualitative manner the utility U of the various alternative production results.

For this reason Val'tukh proposes to use the function U(x) in the formula 4 as the target function Z(x) for the optimalization computations during the economic planning. The loyal reader remembers the columns about the multy-period optimization, based on a intertwined balance. Often the target function Z in those examples is simply the size of the total product. The target function U=C of the formula 4 takes into account much more accurately the true preferences of the planning agency. However a practical disadvantage of this target function is, that she is nonlinear, contrary to the common target functions. That complicates the computations.

Val'tukh has actually performed such computations of a multy-period optimization with an intertwined balance and his target function. The normal needs, the starting point of the computation (x=0), correspond to the situation of the USSR in the year 19679. The perspectivistic needs at the end of the planning period (x=χ) correspond to the consumption of the most prosperous income group of workers and officials, again for the year 1967. Val'tukh divides the intertwined balance into `11 branches, and in this way he can compute the optimal growth path.

This completes the description of the essential ideas of the theory of Val'tukh with regard to social indifference curves. In the previous column about the cardinal marginal utility, which has just been mentioned, the vision of Val'tukh with regard to the historical dynamics of the indifference field has been discussed. Care must be taken to monitor the changes in the indifference curves due to the social development. As long as the field remains unchanged, the selection of the best plan can be represented by a curve in that field, a growth path x(t). With the passage of time t the planned production moves to the right and upwards in the field.

Figure of the utility function
Figure 4: Function η10 / e10×η

Val'tukh remarks, that the simplest growth path is the straight line from x=0 to x=χ. Along this line the quantities change according to the formula xj(t) = η(t) × χj. With the increase of the time t the values of η(t) rise from 0 to at most 1. According to the formula 4 one has on the linear growth path that

(6)     (Πn=1N χn) × ηN / eN×η = C(x(η(t)))

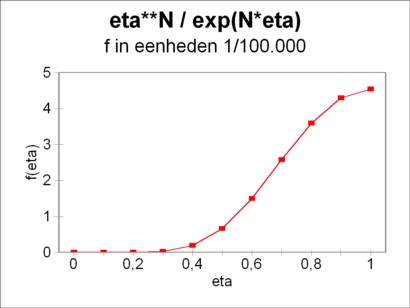
In order to illustrate how C will change along the path, the figure 4 displays the development of the function ηN / eN×η, for the case N=10. The formula 6 can be simply rewritten as follows:

(7)     η = eη × C1/N(x) / (Πn=1N χn)1/N

If desired, next the formula 4 for C can be inserted into the formula 7. This η can be interpreted as a measure for the social progress, and therefore as an alternative utility function. In other words, the formula 7 is a nonlinear transformation from the utility function U=C to the utility function η.

The introduction of the utility function η is interesting, because she leads to a fairly simple expression for the marginal utility10

(8)     ∂η / ∂xj = η × (1 − xj/χj) / (xj × (1 − η) × N)

The interesting aspect about this marginal utility is, that for a given value of xj it increases according as all needs in the situation corresponding to η become more satisfied. For according as one has x→χ, it follows that η→1. Val'tukh calls it a law: the marginal utility of a product j rises, according as the total level of the satisfaction of needs (that is, the quantity η) is raised11. This discovery fits naturally well in the more general picture, which is sketched by Val'tukh, and where the (marginal) utility of a product refers exclusively to the general utility level of the total production12.

  1. De Wolff writes on p.220 of Voor het land van belofte (1954, 1978 reprint SUN): "The posterity will be more just. Certainly also here the time will come, when the marxism is again recognized as the ideological weapon in the liberation struggle of the working class. Then young eyes will read the texts of Van der Wijk and the work, initiated by him, will be extended. His writings will be translated into modern languages, so that it will be accessible for the whole world and the Netherlands will be proud that Van der Wijk has been born and lived here". Nowadays the name of Van der Wijk is attached to the law of Gibrat-Van der Wijk, so that this prophecy has rapidly come true.
    From a historical standpoint it is worth mentioning, that in the years immediately after the Second World-War Harold Ferro, the son-in-law of Van der Wijk, was a pioneer in the research of marketing. Ferro wrote various introductory textbooks, and established his own consultancy agency. She still exists, under the name Ferro Explore!. Thus again a thread is found, that connects the past to the present and to the future. (back)
  2. Already a century ago J.H. Speenhoff sang in the song Ons Hollands ideaal: Then your arm-chair, your cigar / With your wife and the newspaper, / Warm slippers and a gin, / Living in your own house. / That is what we all desire, / That is our Dutch ideal. (back)
  3. This example originates from p.18 in the famous book Theory of games and economic behaviour (1947, Princeton University Press) by J. von Neumann and O. Morgenstern. That book was part of a lot, which your columnist bought at an auction! So everything ends up well. Those who prefer to consult a modern source, can read the chapter 6 in Micro-economic theory (1995, Oxford University Press, Inc.) by Andreu Mas-Colell, Michael D. Whinston and Jerry R. Green. That chapter is devoted entirely to the subject of choice under uncertainty. Your columnist hopes to write a future column about this theme. (back)
  4. Moreover, on p.136 and further in Mikro-ökonomische Theorie (2011, UVK-Verlagsgesellschaft mbH) by W. Hoyer and W. Eibner it is stated, that often the consumption of a product xi requires a certain time ti. Since time is a scarce commodity for any individual, this also limits the utility of quantities. (back)
  5. See p.157 in Micro-economie (1996, Stenfert Kroese), by F.J. Dietz, W.J.M. Heijman, and E.P. Kroese. This introductory textbook is certainly one of the best, and moreover it is written in Dutch. The favourite of your columnist remains Volkswirtschaftslehre (2003, Oldenbourg Wissenschaftsverlag GmbH) by M. Heine and H. Herr. But it must be admitted, that Heine and Herr do not explicitely mention the phenomenon of the utility peak. (back)
  6. Also Bernard van Praag mentions the falling utility on p.97 and further in his book Individual welfare functions and consumer behavior (1968, North-Holland Publishing Company). He even derives by means of mathematics, that the indifference curves can have both the shape of a hyperbola (figure 2a) and an ellipse (figure 2b). For this purpose he develops the utility function u(x0 + h) in a Taylor series around the point x0. Here h represents a vector with an arbitrary small length. It is obvious that in this model the behaviour of the utility function u is determined by the values of the derivates of second order of u with respect to its arguments x. Your columnist hopes to devote a future column to this calculation. (back)
  7. On the other hand it is necessary to remain vigilant against a possible encapsulation by the Leninist rhetoric. It may well be, that from the beginning the planned economy in for instance East-Germany has been built on an alienated and ideologically unsound view. A strong indication in that direction can be found in the book Die Ausnutzung der Ware-Geld-Beziehungen durch die Wirtschaftspolitik der DDR (1961, Verlag Die Wirtschaft). On p.108 and further in that book G. Pfütze argues, that the still existing capitalist (read: private) enterprises in the GDR are much more inefficient than the state enterprises. Their production costs would be much higher. On p.124 Pfütze even calls the private enterprises backward (zurück-geblieben). And on p.123 he discusses the option of centrally fixed market prices: "Sometimes it has been stated, that some want to eliminate the private enterprises with their low productivity by means of a unit price. It is naturally nonsense to introduce a fixed unit price without taking into account the discrepancy between the [low] labour productivity in most private enterprises and the average labour productivity in the socialist [collective state-]enterprises in that branch ... The solution of the discrepancy is only possible ... by a state participation in the private enterprises". Your columnist appreciates state enterprises in situations, where they further an efficient production. But he refuses to believe, that private enterprises are always structurally inferior. Apparently at the time the East-German state has "helped" a little in order to make the private enterprises "unprofitable", to its own detriment. The ideology was more important than reality! Also note the publication date of the book, evidently again an acquisition from the second-hand bookshop Helle Panke. Then there still existed a private sector in the GDR. Later it was almost completely eliminated.
    Those who want to plan, must have solid arguments, and a democratic legitimacy. Wisely Wannes van de Velde sings in the song Het is de mensen niet gegeven (of the record De kleuren van de stad): I do not have power, and that is for the best; / I would not know how or what. / Who knows if I would not bannish / the worthy people to the cold / of a frozen piece of savannah; / in the name of the sacred ideal. (back)
  8. See Entwicklungs-proportionen und Befriedigung der Bedürfnisse (1972, Verlag Die Wirtschaft) by K.K. Val'tukh (in the German language Waltuch). This book was acquired at the Hofbuchhandlung in Löwenberg (East-Germany). (back)
  9. See p.110 and further in Entwicklungs-proportionen und Befriedigung der Bedürfnisse. (back)
  10. See p.132 in Entwicklungs-proportionen und Befriedigung der Bedürfnisse. (back)
  11. See p.135 in Entwicklungs-proportionen und Befriedigung der Bedürfnisse. (back)
  12. Val'tukh contrasts this law of the rising marginal utility with the famous first law of Gossen about the falling marginal utility. He is fairly critical with respect to the first law of Gossen, because it pertains to the utility of one product in isolation. Since in the eyes of your columnist Val'tukh somewhat exaggerates in his criticism, it may be that the cause is found in the ideological struggle between the east and west. The variable η plays a prominent role in the utility theory of Val'tukh. It is a pity, that he does not elaborate on its meaning. (back)